Advanced solving techniques
As seen on Times Online in February 2007
In November 2006, The Times introduced a new extreme level of Sudoku giving players a more difficult level of logic: Super Fiendish. Many have welcomed the new challenge but some have asked for help. So, as we increase the difficulty Super Fiendish, the time has come to present the essential techniques for solving the most difficult Sudoku puzzles.
Please be reassured that all Sudoku puzzles published in the Times are always solvable by logical means. Here we present the techniques that will help you solve the hardest puzzles using only logic and no guesswork.
First a couple of preliminary points: if you have ever completed any Fiendish or Super Fiendish Sudoku, you will be familiar with noting down candidates or pencil marks. Pencil marks are small numbers, usually written at the top of each unsolved square, listing all the possible values for that square. With many advanced techniques, the key is to spot patterns within your pencil marks to eliminate possibilities within other squares. Whenever you solve a square, you must remove that number from the pencil marks in all other squares in the same row, column and block.
The x-wing technique
Essentially, this method uses the fact that in certain cases, there are only two possible ways of placing two numbers in four squares which form a rectangle. There is a relationship between the diagonally opposite squares, hence the “x” in x-wing. The term x-wing itself derives from the x-wing fighters in Star Wars.
The Sudoku in Fig. 1 is partially solved using easy and medium difficulty solving techniques: singles, hidden singles and column/block intersections. Now, no more squares can be solved with these techniques; you are stuck. In the diagram there are complete pencil marks for all squares in rows three and seven, and a necessary column/block intersection to eliminate a 7 pencil mark.
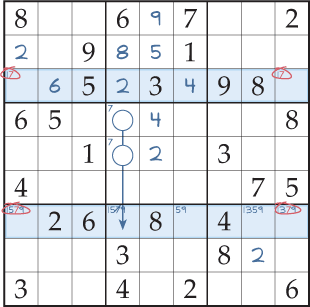 Figure 1
Figure 1
Consider where you might place the 7 on the third and seventh rows (highlighted). You know it must be placed once, and only once, on each of these rows. Here, the only squares with the 7 as a possibility are in the first and last columns. It is a fact that you cannot place the 7 in the first column square of both the highlighted rows at the same time. Neither can you place them both in the last column. Therefore, they must be placed in diagonally opposite corners: one 7 in the first column; one 7 in the last.
So, there are two possible ways of placing the 7; how does this help you solve anything? Well, you have established that the 7 for the first column must be in one of the two highlighted rows; not in any other squares in this column. Now you can cross out the 7 pencil mark from all other squares in the first column. Using the same logic in the last column, you can also eliminate the 7 as a possibility from all squares which are not in either highlighted row (Fig. 2). This leaves one square in the first column with only one pencil mark, so you can now place the 9. Now this square is solved, you can complete the Sudoku using easy methods.
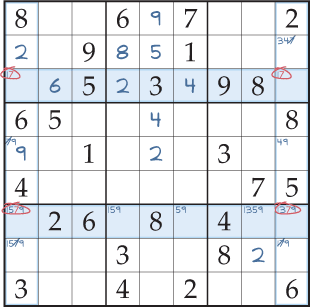 Figure 2
Figure 2
In general, an x-wing is found when for two rows, there are two, and only two, possible squares in which a particular number can be placed, and for both rows these squares lie in the same two columns. In this case, this particular number can be eliminated as a possibility for all other squares in those two columns. Of course, you can exchange rows and columns in this definition.
The Swordfish technique
This is a more difficult method, one of the most complicated for any Sudoku you will find in print. From today onwards, you will find the swordfish technique become a regular feature in the Times2 . The swordfish is similar to the x-wing technique, but on a larger scale, with three rows and columns instead of two. The swordfish technique is named after a World War II biplane called the “Fairey Swordfish”, a Torpedo Spotter Reconnaissance biplane used by the Royal Navy from 1936 to 1945. If lines are drawn along the rows and columns, connecting the squares involved, they can form two rectangles, connected at the corners. This resembles the wings of a biplane, separated by struts.
A swordfish is found when for three rows, there are two or three possible squares in which a particular number can be placed, and for all three rows these squares lie in the same three columns. In this case, this particular number can be eliminated as a possibility for all other squares in those three columns. Of course, you can exchange rows and columns in this definition.
 Figure 3
Figure 3
Fig. 3 is a partially solved Sudoku, using easy and medium difficulty solving techniques; no more squares can be solved using them. To give you a good chance of spotting the swordfish you should enter all the pencil marks in the grid as shown here.
There are no easy routes to spotting the swordfish pattern. So, try focusing on just one number 1-9 in turn as you scan your pencil marks in rows and columns. Look along each of the rows in turn, noting each one that contains only two or three squares with the current number as a possibility. Then, look to see if there are three rows where the squares all fall into three columns. If nothing is found, try again with the columns in place of the rows.
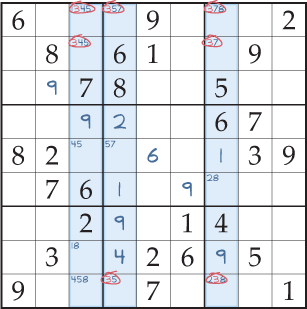 Figure 4
Figure 4
In Fig. 4, the relevant pencil marks are entered to show that you have two possible squares in which the 3 can be placed in the third and fourth columns, and three possible squares in the seventh column (highlighted). Crucially, all of these possible squares lie in only three rows. Therefore, on each of the three intersecting rows, the 3 must be placed in one of the highlighted columns. So, you can eliminate the 3 pencil mark from all other unsolved squares in these intersecting rows (Fig. 5). These eliminations, in addition with a column/block intersection to remove a 7 pencil mark, leave only one pencil mark in the last square in the second row, so you can now solve it to be a 4.
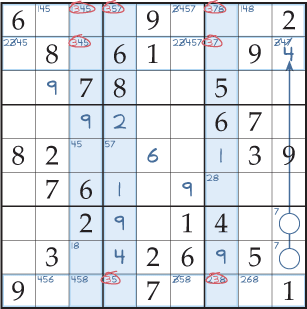 Figure 5
Figure 5
If you like playing some seriously hard Sudoku, try the puzzles at the end of The Times Sudoku 6 book with some requiring the swordfish technique. If you really like Super Fiendish, you will have to wait a little longer for The Times Sudoku 7 with 25 extremely hard Sudoku, many requiring x-wing or swordfish to complete.



